What are Capacitors, and How Do I Use Them?

A capacitor is an electrical component that stores energy as an electric field. A capacitor is constructed with two plates, very close together, with an electrical insulator between them. As a voltage is applied to the plates, an electrical field builds between them. While the field builds, current runs through the capacitor. Then, the current reduces to zero as the capacitor reaches full charge. Capacitance is measured in units of Farads, named in honor of the Scientist Michael Faraday who did a lot of the foundational research in electricity and magnetism.

What are the Different Types of Capacitors?

There are many different types of capacitors. Each of them has their own advantages and disadvantages. The most common types of capacitors are listed in the table below with their key advantages and disadvantages.

Ceramic Capacitors	Very Low Resistance (ESR) Small Capacitances High Voltage Ratings Non-polarized
Aluminum (Electrolytic) Capacitors	Higher Resistance (ESR) Large Capacitances Lower Voltage Ratings Polarized
Aluminum Audio Capacitors	Higher Resistance (ESR) Large Capacitances Lower Voltage Ratings Non-Polarized
Tantalum Capacitors	Low Resistance (ESR) Large Capacitances Lower Voltage Ratings Tends to Catch Fire with Overvoltage Polarized
Tantalum Polymer Capacitors	Low Resistance (ESR) Large Capacitances Lower Voltage Ratings Does Not Catch Fire with Overvoltage Polarized
Supercapacitors/Ultracapacitors	Very Low Resistance (ESR) Very Large Capacitances Very Low Voltage Ratings Polarized

How Do I Use A Capacitor?

You can use capacitors to do two things. Either, store power or to conduct an AC signal. In order to store power, a capacitor is charged to a voltage. Then, the capacitor can be disconnected from the voltage source and used to power something else. One of the most common applications for a capacitor conducting AC power is using a capacitor to bypass power noise to ground, removing it from the circuit.

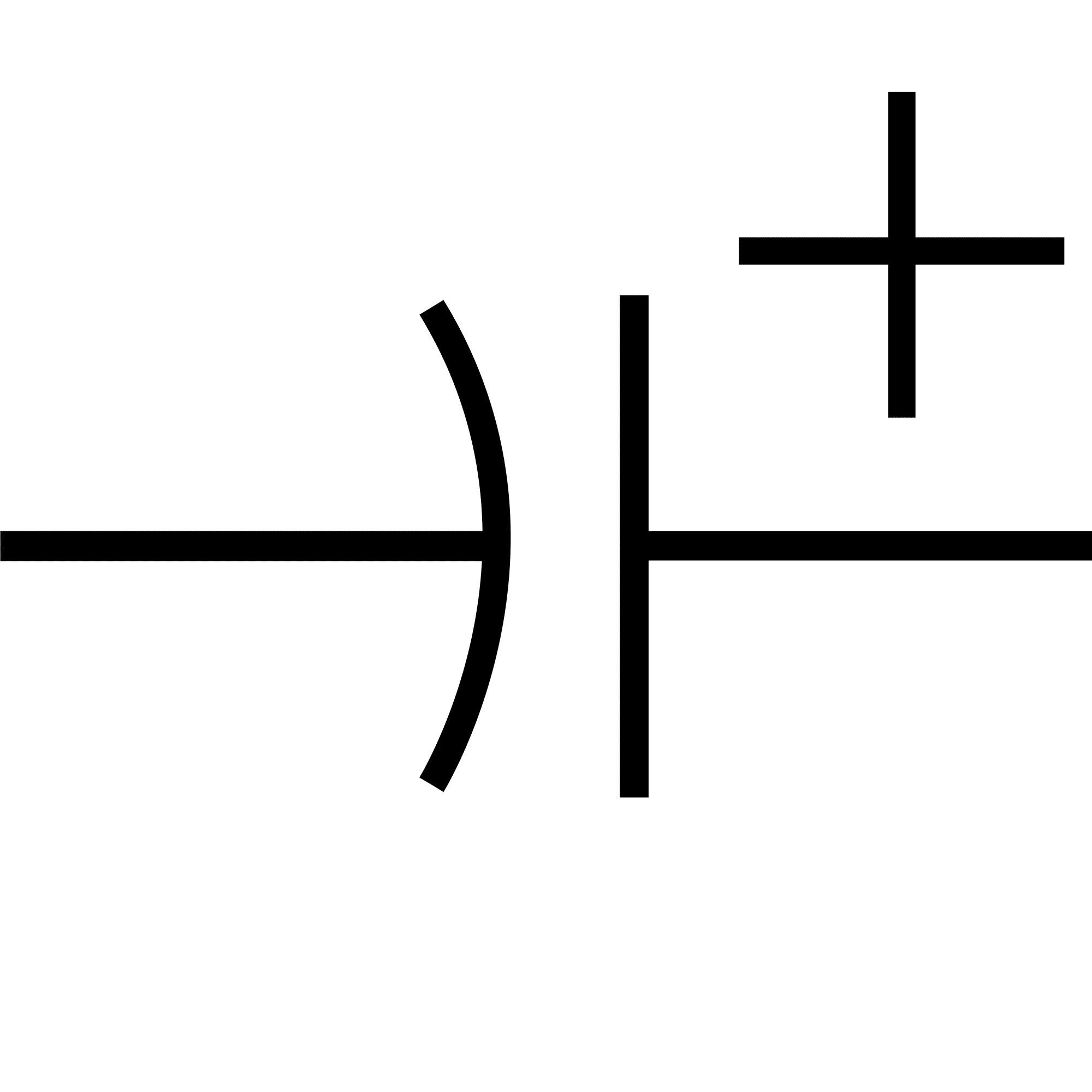
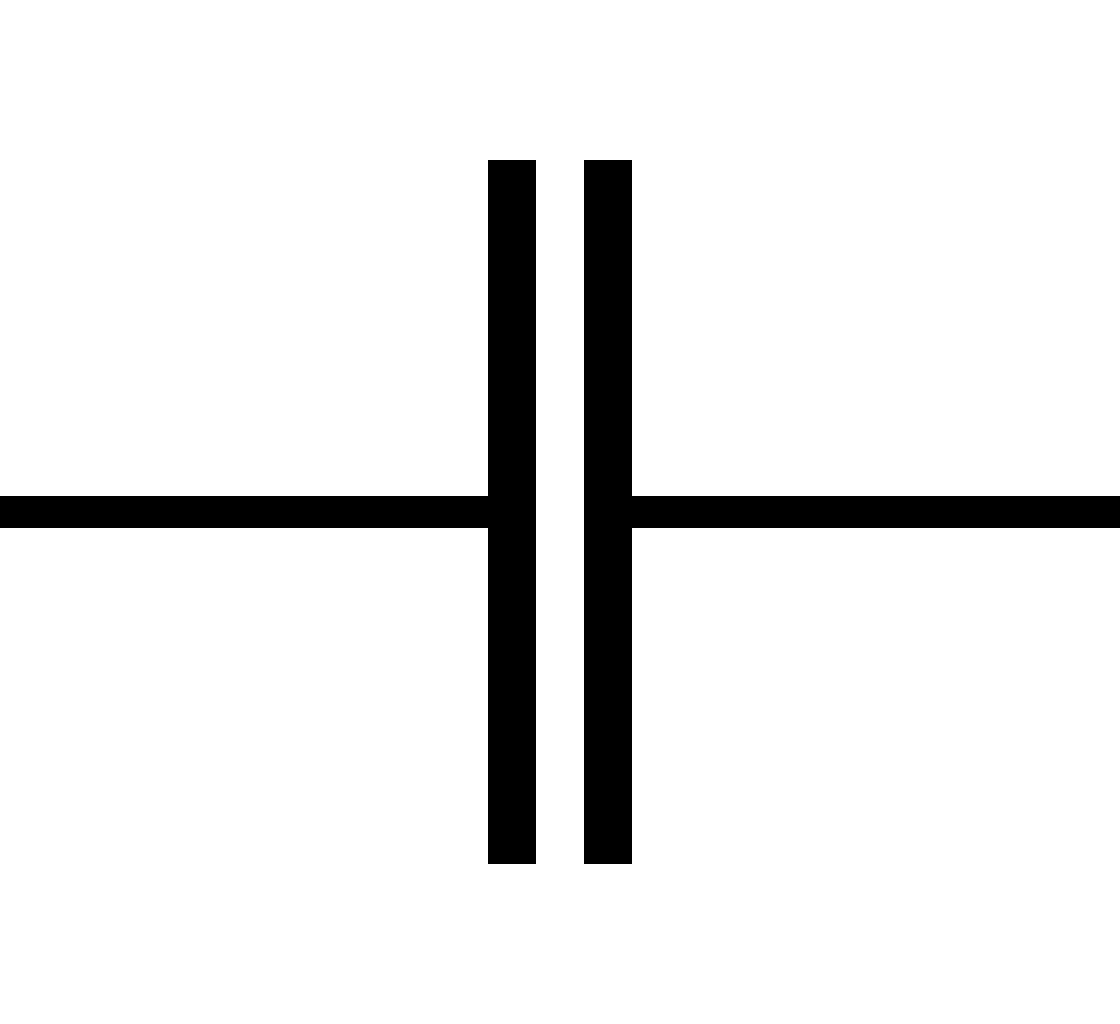
Capacitance

The capacitance of a capacitor is the measure of its ability to store electrical charge. The more energy a capacitor can hold per voltage is its capacitance. This relationship is shown in the equation below.

\(\large C=\frac{Q}{V}\text{ or }Q = CV\)

Energy Stored In a Capacitor

Now, when a voltage is applied across a capacitor, an electric field is generated between the plates, which is stored energy (P). Energy is measured in the units Joules (J). The amount of energy stored in a capacitor is related to the amount of charge in the capacitor, but it is not a linear relationship. When a capacitor is charged electrons collect on the plates inside it.  As the capacitor charges, it becomes more difficult to charge it further because the electrons on the plates push against the new electrons trying to get on the plate.  So, the amount of energy stored per charge increases. Because of this, you’ll notice that the equation to calculate the energy in a capacitor is slightly more complicated than the equation for electrical charge.

\(\large P = \frac{1}{2}\frac{Q^2}{C} = \frac{1}{2}CV^2 = \frac{1}{2}QV\)

Charging a Capacitor

In the same way that it gets harder to charge a capacitor as the charge builds, also the charging slows down. So, if you have a capacitor that is not charged at all and you connect it to a voltage source, it will begin charging as fast as it can and then slow down. The primary limiter is the resistance of the capacitor. Yes, the capacitor has is own resistance or ESR (equivalent series resistance) even though it is not a resistor. This is because, in the real world, everything has some capacitance, resistance, and inductance. So, as the capacitor begins to charge, the rate of charging (current) is given by this equation where R is the total resistance in series with the capacitor:

\(\large I = \frac{V}{R}\)

Now, the reason that the charging slows down are the electrons that have already made their way onto the capacitor’s plates. The force that they push back with is the capacitor’s voltage (V = C/Q). For now, let’s call the voltage from the source V_o and the voltage of the charge on the capacitor V_cap. Now we can take the voltage of the capacitor into account in our charging equation as follows.

\(\large I = \frac{V_o – V_{cap}}{R}\)

So, instantaneously as we connect the capacitor to the source voltage, the charge on the capacitor is 0V. Therefore, the charging is completely uninhibited. But, as soon as some charge gets in the capacitor, the capacitor’s voltage starts pushing back, reducing the charge speed. This relationship results in the rate of charge decaying as time goes on. To represent this mathematically, we use a decay function to show the charging current as a function of time (t) in seconds.

\(\large I(t) = \frac{V_o}{R} e^{ \frac{-t}{\tau} }\)     where the time constant of the capacitor, τ, is     \(\large \tau = RC \)

Similarly, the rise of voltage of the capacitor and the charge on the capacitor can be represented by the growth functions here.

\(\large V(t) = V_o (1 – e^{\frac{-t}{\tau}} )\text{,    } Q(t) = C \times V_o (1 – e^{\frac{-t}{\tau}})\)

Discharging a Capacitor

At this point, I’m sure we’ve dived deeper into the math of capacitors that most of you ever wanted to know. So, I’m just going to write out the equations for a capacitor discharging and we’ll get onto the simulator. The discharge equations are exactly the same, except that the growth functions and decay functions swap.

\(\large V(t) = V_o  e^{\frac{-t}{\tau}}\text{,    } I(t) = \frac{V_o}{R}  e^{\frac{-t}{\tau}}\text{,    } Q(t) = C \times V_o e^{\frac{-t}{\tau}}\)

Try it yourself!

You can see both power storage in a capacitor and AC power passing through a capacitor in the following simulation. Click the switch to charge or discharge the capacitor and watch how the AC noise from the power supply is passed through the capacitor to ground to provide cleaner, DC power to the load. Be sure to notice the charge and discharge curves of current and voltage as you flip the switch.